[CLICK HERE to see the 2d Cellular Automata project in the Labs]
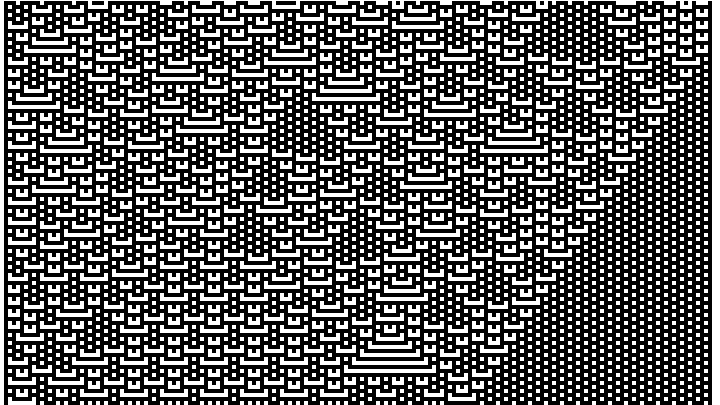
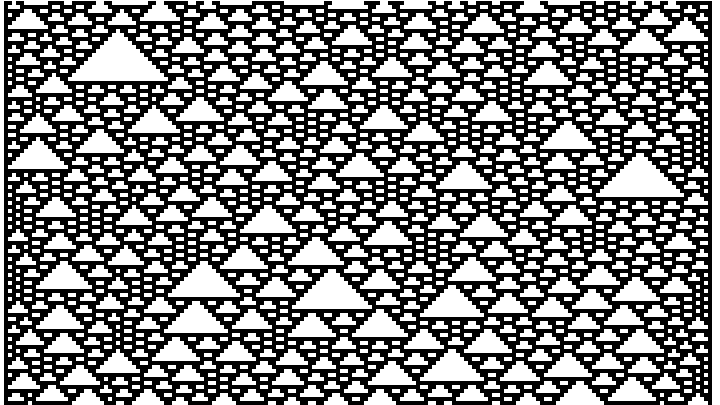
Being over twenty years into my career, and having a little downtime, coupled with the back-to-school energy of September, I have been having the time of my life revisiting old visual and math experiments from the early 2000s. This is one of my favorite – a tool/toy for visualizing 2D cellular automata as described by Stephen Wolfram in his book A New Kind of Science.
I first created this tool in Macromedia Flash back in January of 2003. This was about the time that Actionscript became a useable development language, and it was close enough to Javascript syntax that it was easy to pick up. This was fortunate, as my career for the next seven years depended entirely on my Actionscript programming skills. Then I took a sharp left turn into Drupal, about which the less said, the better.
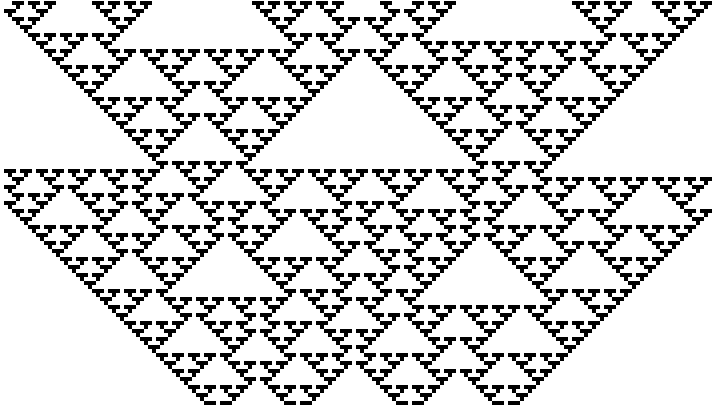
The patterns are created by iterating through an array of ones and zeroes, and choosing the pattern of the next line by applying simple rules to the cells of the previous iteration. As with the earlier Javascript projects, you can save interesting patterns as a .png by right-clicking on the canvas.

I put this together in my spare moments on Codepen.io. You can see the pen with fully-editable code here.